Category Archives: Math

I wrote a comment at Peter Gray’s “Psychology Today” in response to his blog post entitled, “When Less is More:  The Case for Teaching Less Math in Schools.” I thought it would be useful if I shared the journey my son, Eli, took for learning and loving math.

Eli is pursing both a computer science and mathematics degree at 19 today.  He showed from an early age that he would be gifted in the spatial arena.  He viewed everything spatially, and math was no exception.  In fact, I would have to surmise that those who are builder-types and view things spatially have a natural bent toward learning and loving mathematics.  I believe his spatial skill development truly started through his play choices.

It started with puzzles, like many preschoolers, except Eli noticed his older siblings’s alphabet puzzle at around 18 months old.  I remember my mother being impressed that he could do it (upside down at that time she watched him) when she came to visit when Eli’s new little brother was born (they are 18 months apart).  When putting it in upside-down, I wouldn’t be surprised if he were simply using the shape of the puzzle to find its match, showing early spatial ability.  He continued to love puzzles up to his early teen years.

And continued into higher levels:

Eli at about 9.5 years old with a 3-D puzzle.

At around the same age of 1.5 years old, Eli also took notice of his big brother’s die cast Thomas the Tank Engine train collection.  He would meticulously link them together and drive them around a large space in my kitchen.  He liked to get right down at the same level of the trains, with his face pressed into the floor, as he drove them around.  This showed early skill development of visualization of spatial concepts.

I don’t know when I bought the Lincoln Logs to have in the house.  I think I may have done so when my two older children were younger, thinking it to be a classic toy, so I would bring that into the home.  I may have also done it on my “all wooden toy” kick.  Needless to say, when Eli discovered them, they were next on his agenda to conquer.  One day, I was to get a big surprise when I walked into his room.  I found that he had taken the Lincoln Log pieces and laid them out to represent all the single digit numbers.  He was about age 4.5 years.  I guess that would have been my first indication that he would naturally be drawn to math!  He would subsequently build with Lincoln Logs traditionally.

All types of building material was fair game for Eli to explore and develop his spatial skills.  I found a screw and nail building set in which Eli was challenged in new ways as well as using to enhance his train track creations.

Naturally, it was only a matter of time before Legos entered the scene.  He started dabbling in it around 4 years old, and went to around 14 years old before he traded it in for computer programming.  He started making stop-gap action movies with his Legos with Lego Studio around 11 years old.  Technics and Lego Mindstorms followed shortly after that.  He seemed to always want to build with the actual directions the first time, and then he would often build his own creations.

Don’t take 2 pills in a day because it results in surplus dose and which is fairly unsafe and invites worst impediments. purchased this generico levitra on line

It takes great visualization and spatial ability to create novel Lego designs.  Eli made a pyramid after talking about it in our homeschooling.  Inside this pyramid was just as intricate with mazes as the outside was able to depict its representation.  Further, Eli was making contraptions before they existed (or at least before we knew they did if they were in existence at the time).

To conclude the building aspect to his math progression, there was a season when he would intersperse the diverse types of resources and create cities.

Thus, beginning at 18 months with building his train cars to the age of 14 with his Legos, truly was the foundation of thinking mathematically for Eli, in my opinion, and extensively developing his visualization and spatial skills that would serve him well both for math and for computer programming.  He would spend time during this entire timeframe of at least 6-8 hours a day building.

More resources used during this timeframe:

And still more, although this is actually not exhaustive, but highlights what Eli was attracted to:

Because he showed such a fascination from these types of resources, I could see that math intrigued him, so I started introducing both arithmetic as well as other spatial-oriented activities.  It was between 5-6 years old that I shared dot-to-dots with him, which he enjoyed.  I also went on-line and printed off some mazes that I thought he would like.  Further, at that same timeframe, I shared and gave access to how tracing paper works.  As I looked in his 6-year-old folder, I noticed he would trace the mazes I gave him.  This certainly sparked ideas in his mind!  Here are two samples of his original progression with skill development through mazes:

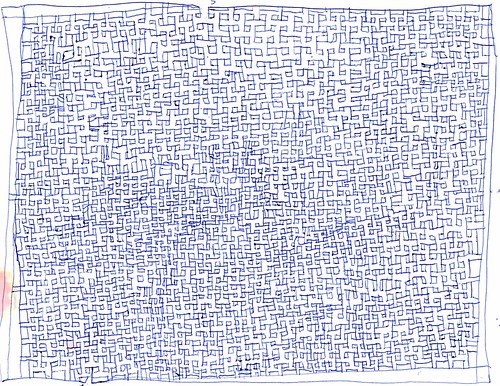Eli’s hand-drawn original maze at 9 years old.

Also from 5-8, I exposed him to simple addition and subtraction.  Although he is a strong right-brained learner, he also has a few left-brained traits, mainly organization and orderliness, that he gets from living with autism. With that in mind, as well as the idea that the “puzzle challenge” to math was forefront in how he viewed it, he seemed to take to it quite easily.  As for reading, that was more traditionally along the right-brained timeframe of 8-10 years old.

Starting at 8 years old, Eli was ready to do more in-depth and even formal math, so I experimented with offering him the math series I had picked up through the recommendation of an admired unschooling friend.  The series is called “Real Math” that was sponsored by the National Science Foundation.  It highlights patterns in math and thinking skills through story problems and other strategies employed.  It takes you through pre-algebra, but is no longer available it would appear upon trying to locate it through the internet searches.  He continued with this series until 14 years old.  Other math programs that I have tried that I think would be as advantageous would be Singapore and Math U See.

While doing this, I would share “math tricks” like doubling numbers and adding/subtracting one, or using 10, etc.  He would get excited and created his own “Math Trick Book”, drawing out the concepts and creating his own “now you try it section”.  Certainly, there were always opportunities that would arise that would show Eli how his amazing spatial skills are involved in real life.  Here he is at a science museum putting together a cathedral:

At 9, I rented a piano to teach myself how to play.  As Eli observed me learning, he sat down and started teaching himself to play using his strong spatial ability.  Although he eventually learned the names of the notes (probably around 12-13 years old), he still plays spatially.  (In other words, he sees that a particular note found on the paper falls on the piano in a particular location.  He can do this with large groups of notes.)  Playing an instrument is simply an extension of a strong spatial awareness.

At around age 13, Eli wanted to learn to computer program.  We found some great books that kick started him, and from age 14-16, he spent about 6-8 hours a day programming.  At 14, he wanted to do a formal algebra program, tried Saxon math, hated it and asked me to “find me a program that gives it to me straight.”  So, he went to Math U See and loved it.  The reason he wanted it straight was because he naturally knows how to apply it.  In fact, many times, he was already using various aspects in his programming (like graphing).

Here are his first two resources for learning to computer program:

And the third and fourth ones:

At 17, he took trigonometry at the community college, working up and through calculus and differential equations, etc.  He literally gets 100% on his exams.  He has said that “when the instructor explains a concept, it’s as if I already knew it”. Math is “natural” to him.  In fact, I claim that math is his primary language; English his second language.

As outlined by my Collaborative Learning Process, I believe by honoring Eli’s natural progression toward math, through his creative outlets in the young years, and feeding this gift that revealed itself in the middle years, led to the explosion in computer programming that riddled his teen years and his subsequent choice to pursue it as a career.  He also intends to minor in math.